Back in 2014, STATS Inc. introduced the notion of gravity, which was explained by Kevin Pelton as a series of two measures: gravity score and distraction score. In Pelton’s explanation, gravity score measures the relative distance a primary defender strays from their offensive counterpart and distraction score quantifies the amount of attention that same defender gives to the ball handler. In one of the worst episodes of self-reference, Tom Haberstroh piggy-backs on Pelton’s description by developing a measure called respect rating (Pelton’s article references Haberstroh’s article; which in turn references Pelton’s article… they were published the same day). While the two articles are incredibly vague in describing the mechanics of gravity, we were given an insight into some analytics that can be developed from tracking data.
To give a simple overview, gravity is a measure that ranges from 0 to 100. It reflects relative distances between one defender and two specific players on the court: the ball handler and the offensive player being guarded. The idea is an attempt to quantify the “Man-You-Ball” defensive posture a player takes on the court. The tighter a defender guards an offensive player, the higher the gravity. The tighter a defender moves towards the ball, the more distraction they observe from guarding their man. The idea is very straightforward.
In this article, we serve up an introduction into gravity from an elementary point of view: center of masses. We will use this article as a springboard into understanding the role gravity in areas such as hedging, switching, pre-switching, and ultimately, spacing. Commonly, gravity is seen as a measurement of defensive players relative to shooters; however, it is much much more than that.
Our goal here is not to replicate gravity, but rather give a relative definition of gravity that captures the original intention of gravity while giving us a tool set to start quantifying difficult features using spatial statistics.
Introduction: Fixed Gravity, Two Bodies
During my NCAA playing days, we were taught the 1/3-1/2 rule; which meant we guard our man one-third of the distance from our man when he is one pass away and 1/2 the distance away when he is two or more passes away. This idea (now nearly 20 years old; and was even taught earlier) is simply fixed gravity.

Red defender playing one-third distance of on one-pass player.
In this case, we identify the two sources of gravity: the ball handler and the off-ball player a defender is guarding.

Included labels of “Gravity” and “Distraction.” However, both are just two gravity terms.
However, if we merely drag along this line, we run the risk of one of the simplest plays in the book, the backdoor cut towards the basket.
This means that the defender has the potential to get burned almost every time as they are not protecting the basket when they are the lone responsible player for protecting the basket.
Note: There are situations where a defender may refuse to protect the basket, such as in BLUE defense, where the primary defender’s responsibility is to refuse penetration into the key; and a secondary defender is responsible for covering a driving lane to the basket, thus protecting the rim.
Basket Gravity: Fixed, Three Bodies
To reduce the probability of a backdoor cut, we focus on sagging towards the basket. In this instance, we have now defined a third gravitational pull. If we consider a simple constant model for this, let’s pick a naive number and suggest one-sixth the distance to the basket.What happens to the defender now?
First, the defender is no longer on the vector between the two offensive players. Instead, they are pulled in the direction of the basket, contained in the triangle formed by the two offensive players and the basket.
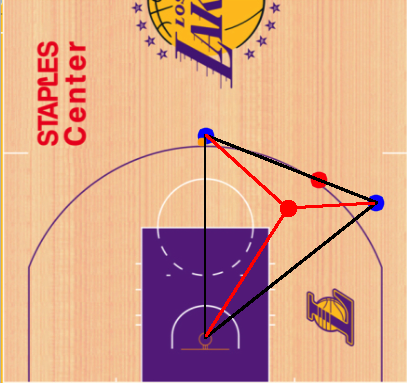
The black triangle formed by the offense and the basket is the region for which the defender is most likely going to operate in.
When the defender sags, he forms three new triangular regions. These are indicated in red. The upper triangle between his man and the ball handler is the original gravity-distraction region, which we call contest zone. The right-hand triangle between his man and the basket is the recovery zone. The left -hand triangle between the ball-handler and the basket is the help zone. The lengths of each of the red spokes identify the distances to each of the three gravitational bodies
By assuming the fixed gravity model, we merely need to find the position that gives us the one-third and one-sixth distances we require. This calculation is commonly known as an n-body center of mass. In the n-body center of mass, we effectively calculate

Here, p_i, are the positions of offensive player (or basket), i, on the court. The values, m_i, are their associated masses, or weights. In our fixed gravity construct above, we have this one-third, one-sixth rule. Under this construction, we set the mass of the offensive player to one and see that the masses of the ball-handler and basket become 1/2 and 1/5, respectively. This gives the explicit solution below.

Explicit solution of of the one-third, one-sixth fixed gravity model.
In motion we can see the backdoor cut become guarded.
Fixed Gravity: More Bodies
The description above is a classic denial defensive drill for wing defenders, where the ball-handler is a teammate throwing the ball into play. In reality, there are more players on the court and this begins to complicate even the fixed gravity model.
For instance, let’s place in one more offensive player and a two more defensive players.

We solved three fixed gravity center of masses.
Here, nothing has drastically changed. We isolated each part of the defense and solved the center of mass problems with respect to the gravity rules. The sketch is nothing out of the ordinary.
If we extend to the Minnesota Timberwolves offensive set, we can see how this fixed gravity problem adjusts the defense.

Defense (red) using fixed gravity model of defense against the standard Timberwolves offensive pin down PnR set.
We see that the defense looks a little compacted, and somewhat unrealistic. However, the basic fundamentals of the defensive scheme are preserved: one-third distance from man to ball, one-sixth distance from man to basket.
And now as a play goes into motion, we are able to capture the intricacies of the movement. Pay close attention to the screen. This becomes important.
As we can see in the optimal movement, the defender caught in the pin down must manage to go under the screen and effectively beat his man to the pick and roll screen. This requires first that all screens must be avoided and then that all defenders are perfect at anticipation.
Varying, Constant Gravity
In the introductory cases above, we assumed that all offensive players have the same gravity. We know this is not true. We know that Stephen Curry needs to be closely covered on the perimeter more often than Tristan Thompson. In this case, we are able to start changing the one-third, one-sixth rule from above to a vector of gravity values.
In this case, Curry can have stronger gravity than Thompson. In the case of Curry, we may see the one-third rule get tightened up to one-sixth. Similarly, we may see Tristan Thompson move from one-third to one-half.
If we are able to measure these masses/weights, we are then able to start making decisions about how to guard a possession. For instance a wing screen with a low gravity screener may give the defense the opportunity to either BLUE the screen, leaving a Tristan Thompson-type player to be the perimeter shooter; or trap the ball handler off the screen.
Making such decisions on defense requires the entire defense to react (or at least two members). To start to understand these decisions, we need to look into the interaction of defensive players.
Interactions: Screening, Switching, and Helping
Up to this point, we focused on the most rigid form of gravity that was depicted as three-body action. That is, all players have the same gravity, that gravity is constant, and gravity depends only on the Man-You-Ball with Between Your Man and the Basket philosophies.
In reality, gravity of players is not a one-way street. Applying Newton’s third law of forces, we find that defensive players contribute equal and opposite gravity onto the offense. Let’s illustrate this:

Initial Offensive Set for the Minnesota Timberwolves
If we look at the action in the video above, starting by the initial Timberwolves offensive set above; we know that a pin down screen occurs to set up a pick-and-roll action. This means that two defensive players exhibit gravity!

Two defenders are exhibiting gravity!
This defender driven gravity leads to the simplest defensive interaction on the court: the switch. Two players may switch in these exchanges. What this indicates is that the defender releases gravity on their primary man and picks up the new offensive player. In the physical setting the defender on the switch “orbits” the first offensive player and then gets pulled into the “orbit” of the second offensive player. Therefore a screen is measured by the amount of third force law interaction.
Finally, if an offensive player has high gravity, let’s suppose this is LeBron James in the post, then other defenders may hedge in the direction of James in attempt to reduce the probability of scoring on the possession.
In a more concrete example, let’s consider the BLUE defense. In this case, a defense may blitz the pick-and-roll. When the blitz occurs, a defender pulls across the lane in a two-nine attack to influence the baseline drive of the ball-handler from attacking the rim. This means, we may end up with three defenders on two offensive players for a short period of time. More importantly, this suggests that the ball-handler has a high amount of gravity for this brief period of time.
The question is then, how does the gravity of a player change over time?
Making Gravity Fluid
Now that are are able to model hedging, screening, and switching, we can now start to ask how to compute the gravity for a player. Above, we assumed that the gravity of each player was fixed, constant over time, and results in a center of mass calculation for positioning defenders. In reality, gravity is fluid. That is, it changes as players move on the court.
A simple example would be: How close is a center guarded when they are 85 feet from the basket? In this case, this is simple transition. The reality is, the center is not guarded. Instead they are met down-court, if possible. But once that center comes into the half-court offense, their gravity increases. As they get closer to the rim, their gravity may increase even more.
Given this example, we need to identify a method for measuring gravity. Here’s one method: N-body solutions. Recall that the center of mass solution, given a set of defensive requirements (protect the rim, deny passes) realizes gravity as masses. The N-Body problem is the equilibrium of gravity when all masses are are put into play.
What this means is, applying Newton’s three force laws, we should have 55 combinations of masses: 5 defenders, 5 offensive players, and one basket. Since we are able to measure all 11 positions, q, on the court, we can write the N-Body problem as

When this quantity equals zero, we have equilibrium. The values of m are the masses we are interested in. The value G? This is merely a scaling factor.
One Type of Solution
One method for estimating gravity in this situation is to break up possessions and compute the N-Body problem. Over the course of the possession, we can take the collections of masses for players and yield a response given by points scored during the possession.
Here, we are able to create a model that uses the estimated masses at each time stamp, with a class label of points scored.
Note that despite having 55 combination of masses at each time step, we only obtain 11 masses for the gravity calculations. What this results in is a N x 2P x Q x R x S x Z tensor with the entry of the computed mass. Let’s break down this tensor…
N: The maximal number of time steps in a possession. Will be sparse with structural zeros as possessions tend to be 12-14 seconds in duration; for 300 – 350 time steps. However, if we take the maximal possession (say it is 40 seconds), this value is 1000 entries.
P: The number of players in the league. We select 2 as there is an offensive gravity as well as a defensive gravity for each player. This means P is typically near 950.
Q: Finite baseline index. As the baseline is fifty feet long, a binning of the baseline into 2 foot segments, we obtain Q to be 25.
R: Finite sideline index. As the baseline is ninety-four feet long, a binning of the sideline into 2 foot segments, we obtain R to be 47.
S: Class of possession scored. Only two possible values.
Z: The number possessions.
This results in a tensor that is LARGE, but also SPARSE. The non-zero elements are the estimated weights. Stacking these weights in this manner, we obtain a description of offenses and defenses relative to the locations on the court over the course of the possession.
More importantly, we have a feature space that we can finally create a model. One such model may be a neural network or a support vector machine. Or more importantly, if we are interested in spacing, we can look at the CANDECOMP-PARAFAC Decomposition that yields information on gravity values associated to particular locations on the court that lead to scoring opportunities. And more importantly, identifies defenders that have weak gravity, indicating over-confidence in their defensive capabilities.
Pingback: Gravity Example: 0.04s of Computation | Squared Statistics: Understanding Basketball Analytics
Pingback: Weekly Sports Analytics News Roundup - December 26th, 2017 - StatSheetStuffer
What a fantastic read on Data Science. This has helped me understand a lot in Data Science course. Please keep sharing similar write ups on Data Science. Guys if you are keen to know more on Data Science, must check this wonderful Data Science tutorial and i’m sure you will enjoy learning on Data Science training.:-https://www.youtube.com/watch?v=Y92koZVlgzs
LikeLike